Measurement Notes
The best overview of how to measure trees is provided in the document "The Tree measuring Guidelines of the Eastern Native Tree Society" written by Will Blozan and can be found at the Eastern Native Tree Society website: tree_measuring_guidelines.htm For tree height measurements and girth measurements the same starting point on the ground is used. They are both measured relative to where the tree first sprouted. If on a slope that point is where the pith or center of the tree intersects the ground surface - from mid-slope on the ground surface. On level ground be sure to measure from the actual base of the tree rather than from the debris pile that often mounds atop the root surface - look for the low point in the notches between the exposed roots.
CBH
Circumference at breast height - Also known as CBH, this measurement is made in inches at a point on the tree trunk, 4 1/2 feet above the ground. If the tree is growing on a slope, the 4 1/2 feet is determined at mid slope. This is the point halfway between the high and low points where the trunk meets the soil. If the tree is multi-stemmed, meaning it has more than one pith at ground level, then largest leader or trunk is measured at a height of 4 1/2 feet. If fused multiple trunks extend above the 4 1/2 foot height, then the girth should be measured above the height of the fusion.
For a single stemmed tree that branches below 4 1/2 feet, the girth should be measured at the narrowest point below the lower most significant fork of the tree noting the height of the measurement. If the tree has abnormal swelling, burl, or other abnormality, the measurement is to be taken at the narrowest point below 4 1/2 feet, and the height of measurement is to be noted. Use reasonable sense when measuring these types of trees as occasionally a measurement just above the 4 1/2 foot height might be more appropriate. In all cases the girth is taken perpendicular to the axis of the trunk, not parallel to the soil. Measured girth is the best approximation of size, since it is a real number, without the built in assumptions of circularity used to calculate a diameter. Even girth has its limitations, as a sinewy or contorted trunk will have of hollows and ridges that are not accounted for in the measurements. Diameters calculated from such trees will be overstated
Tree Height
Tree Height is the most difficult of the three parameters to measure accurately. The two biggest sources of error are misidentifying what is the actual top of the tree, and the fact that the tree top may not be directly over the base of the tree. An analysis of 1800 tree measurements by Bob Leverett found that the average offset of the top of the tree in the direction of measurement for the entire dataset was 8.2 feet (representing and actual offset of around 13 feet). What does that mean in practical terms? If you were measuring a 1o0 foot tall tree at an angle of 45 degrees, a 1:1 slope, then this offset alone would lead to an error of 11.6 feet.
The Eastern Native Tree Society measurement technique involves the use of a laser rangefinder to directly measure the distance to the top of the tree and to the bottom of the tree. With these numbers and a little trigonometry the actual vertical height from the top of the tree to a horizontal plane and the actual vertical height from the base of the tree to the same horizontal plane can be calculated. It doesn't matter if the top of the tree is directly over the base of the tree. In addition the crown of the tree can be explored to find what is the actual top of the tree and not a high looking branch on the front of the tree. Tree heights can be measured with these techniques to within a few inches. However the downside of using these methods is the cost of the instruments needed to do the measurements.
For most people wanting to measure a tree the interest is more casual. An accurate measurement is wanted, but without the expense of several hundred dollars of instruments. To get accurate measurements some care must be taken. First try to view the tree from several different angles to see where the actual top of the tree is located. Use that point for the measurements. This will eliminate the greatest potential for error. The second step you can take is to see if the top of the tree is offset from the base of the tree. If you can determine the point directly below the top of the tree use that distance when when making your distance measurements. The stick method requires only a straight stick or yardstick and a tape to measure the distance along the ground to the base of the tree.

First, measure the distance from your eye to the joint of your thumb and index finger (arm is stretched out). Next, hold the stick straight up and down at arm's length in front of you and make sure the portion above your hand is the same as what you measured from your eye to your hand. Step backwards until the tree's base appears to rest on the top of your fist, while the top of the stick appears to touch the top of the tree. At this exact point, the height of the tree is equal to the distance from the base of the tree to you. Place a stake in the ground and measure (in feet) from the trunk of the tree to the stake to find the height!
The stick method works because of the trigonometric principle of similar triangles. The hand-stick-eye triangle is proportional in size to the base of the tree-the height of the tree-distance to the tree triangle. In the illustration above of the stick method, the measurer's arm is shown being held straight out in front of them, with the distance from the users eye equal to the height of the stick above the hand. Using this pattern the distance to the tree is the same as the height of the tree - no math is involved. However it is not always easy or possible to get a good shot on level ground to measure the tree height and if as you hold the yardstick and move your hand up and down the distance from your hand to your eye changes.
The same procedure can be used with a few measurements and some basic multiplication. So long as the yardstick is held straight up and down, the various measurements are still proportional. The ratio of (the height of the stick above your hand to the distance from your hand to your eye) is the same as ratio of (the height of the tree is to your distance from the tree.) Therefore if you measure the height of the stick above your hand (in inches), the distance from your hand to your eye (in inches), and the distance from your position to the base of the tree (in feet) you can calculate the height of the tree. This can be written as a simple formula:
(length of stick x distance to the tree)/(distance to eye) = Tree Height
Using this simple formula the height of the tree can be calculated no matter what angle you are holding your arm, and no matter what the length of the yardstick that extends above your hand. This has a big advantage if you are measuring a tree on uneven ground or if you can only measure the tree from a single angle. One problem that also often occurs is that to actually see the top of the tree, the measurer must be farther away from the tree than possible using a yardstick length of 23-25 inches (average arm to eye length). Using the simple formula above a smaller length of yardstick can be used allowing the measurer to actually see the top of the tree. This is an excellent low-tech method to measure tree height.
The most common method used is to measure height with a clinometer at a distance of 100 feet. At this distance the tree height can be directly read from a percentage scale on the clinometer. The procedure is repeated for the base of the tree to get the height between eye level and the base of the tree. If the base of the tree is below eye level, the two heights are added to get the total tree height. If the eye is below the base of the tree, the eye to base height is subtracted from the eye to top of crown height to get actual tree height. This is fine for many trees, but has some limitations. This basic methodology can't be used unless the line of sight to the base of the tree is fairly level. With increasing angles the actual horizontal distance to the tree becomes increasingly smaller than the taped distance to the base of the tree. This can be corrected with some math, but this step is often ignored. A second and more significant problem is that from a distance of 100 feet, the tops of many trees can't be seen from the ground. With this perspective often branches on the front side of the tree are mistaken for the tree top leading to major busts in the tree height calculations. One way to avoid this problem is to measure tall trees, or trees with flatter crowns from a greater distance away. At 150 feet from the tree, the readings from the scale would be multiplied by 1.5 to calculate height, from 200 feet the percentage readings on the scale would need to be multiplied by 2 to calculate tree height. Just because a more hi-tech instrument is being used does not mean the readings will necessarily be more accurate. If the angle to the base is steeper, and if the user doesn't have a laser to measure the eye level distance to the trunk, this method can still be used, but with some modifications and trigonometry. If the angle between the observer and the base of the tree is more than a few degrees, then it is better to use the degree scale on the clinometer. This method requires some basic trigonometric calculations that can be handled by a $10 calculator. Three numbers must be measured 1) taped distance from the eye to the base of the tree, 2) angle up or down in degrees from the eye to the base of the tree - call this angle alpha, and 3) angle from the eye to top of the tree - call this angle beta. The change in height either above or below the eye level to the base of the tree is sine(alpha) x taped distance. At this point the true horizontal distance on a level line to the tree must be calculated. The horizontal distance to the tree is cosine(alpha) x taped distance. Write this number down as it is needed to calculate the total height from a horizontal line to the top of the tree. The height from eye level to the top of the tree is tangent(beta) x horizontal distance. If the base of the tree is below eye level, adding these two height measurements together will give a total tree height. If the base of the tree is above eye level, subtracting the height to the base of the tree from the height to the top will give a total tree height. This method still assumes that the top of the crown is directly over the base of the tree. Also if the observer is not far enough away from the tree, it is easy to mistake a forward slanting branch for the true top of the tree. Cross-triangulation: If the top of the tree is not directly over the base of the tree, then could you locate the point directly under the tree-top and use that point for measurements? Yes, you can, but it is not easy. Let's re-examine the idea of cross triangulation to locate the point on the ground directly below the top of the tree.
To locate the position of the top of the tree you must sight the top of the tree from two different positions. First walk around the tree at a distance and locate the highest point of the crown. It is easier to triangulate the top of the tree if there are two people. Use a plumb-bob, essentially any string with a weight at the bottom. Sight with the string the top of the tree and the corresponding line on the ground. Run a line - the tape works well - along the ground along the line of sight under the top of the tree. From a second position at a approximate angle of 90 degrees from the first (right angle to the side), sight the top of the tree and the ground using the plumb-bob. Have the second person walk along the marked line on the ground until he is in line with the top of the tree as viewed from this second direction. That point should be the point directly under the top of the tree. Be sure to mark this point. Walk around the tree with the assistant standing at this point. If you are actually under the topmost point of the tree, he will appear to be directly under the top at all viewing angles. With the projection of the tree top on the ground marked, find a position where you can see top of the tree, the bottom of the tree, and the point below the top of the tree. Follow the procedures described before to measure the distance the base of the tree is above or below a level line from your measurement point. To reiterate: Measure the angle in degrees from your eye level to the base of the tree. Mark this angle in your notes. Then stretch a tape from your eye level to the base of the tree. You want to measure the distance to the side of the tree, because you really want the distance to the center of the tree, not to the front of the tree. The height of the base of the tree above or below the level line is equal to sin (angle) x taped distance. From the same point measure the angle to the top of the tree. Mark this down. Now you need to measure the horizontal distance from the measurement position to the projection of the top of the tree on the ground. If the slope is not great, it may be possible to simply stretch the tape horizontally between the positions. You don't actually need to measure the point on the ground, because you are not interested in the vertical position of the point on the ground, just its horizontal position. If you can't stretch the tape horizontally, then measure the point as you would the base of the tree above. You can use the point on the ground, or shoot to your assistants belt buckle, or the top of his head, just so long as you measure the angle and stretch the tape to the same target point. The horizontal offset of this point is cos (angle) x taped distance. Write these numbers down, so that you can check your calculation later for confirmation. Now the height of the top of the tree above the horizontal plane is equal to tan (angle to the top) x calculated horizontal distance to the top's projected position on the ground. The height of the tree is the sum of the vertical distance above or below the horizontal plane to the base of the tree, and the height of the top of the tree above the horizontal plane. Are the numbers good? They can be. It is difficult to accurately locate the position of the top on the ground, so this introduces a potential error. The errors in the clinometer readings are still potentially there. These potential errors are likely relatively small. The biggest difficulty is the time it requires to do the cross triangulation. When using the ENTS methodology for measuring trees with a laser rangefinder and clinometer, the process of cross triangulation is not needed. You can literally walk through the forest checking out trees as you move and get a fair approximation of their height. When you find a tall tree, its height can be calculated in a matter of minutes, as opposed to an hour or so to do the cross triangulation. Much more forest can be surveyed. In addition, in rough terrain, or in areas with thick undergrowth or numerous trees, it may not be physically possible to do a cross-triangulation to determine the ground position of the tree top. In some cases the person making the measurement will not be able to see both the top of the tree and its base from the same position. Measuring relative to a shared intermediate point is fairly easy to do with the laser rangefinder and clinometer, but is hideously difficult to do using cross triangulation methods. And finally, a single person can measure a tree using a rangefinder and clinometer, and while it is possible it is difficult for a single person to use the cross-triangulation method.
Crown Spread
The last two measurements needed are for average crown spread. This is a horizontal measurement, from leaf tip to leaf tip, of the shortest spread, and the longest spread of the tree through the main mass of the tree canopy. Adding the two numbers together, and then dividing by two will give you the average crown spread.
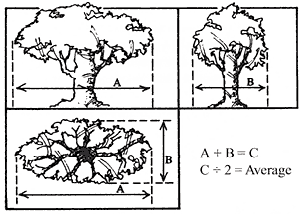
The Maryland Big Tree program collects both average crown spread information for use in the American Forests Big Tree Program and maximum crown spread for the Maryland big tree list.
Measuring crown spread is difficult on trees where the branches are high off the ground. If two people are present, each should walk to where the tips of the farthest branch tips are directly overhead, then measure the distance between the two points at ground level. It is often hard to estimate when a branch tip is directly overhead. If you have a clinometer use it to determine 90 degrees. Otherwise use your best estimate. Someone viewing the tree and you from the side may be able to help align your position to better locate the branch tip.
Good Luck with your tree measurements, and we hope to see your submissions to the Maryland Big Tree Program.
Stick Method and Crown Spread diagrams courtesy of Ohio Big Tree Program